Day Lab Part 1: Angular Diameter of the Sun

The Astronomical Unit (abbreviated AU) is roughly the distance from the Earth to the Sun and is defined as 14,959,787,070,000( approximately \(1.5\:\times\:10^{13}\)) centimeters in length. The AU is an important unit of distance in astronomy and is used to evaluate stellar system scale distances. Additionally, the parsec is defined in terms of stellar units. Clearly this is an important unit in the study of astronomy, but how would we be able to derive that large number if we weren't given it? We set out to answer exactly that question in our day lab for Astronomy 16. Along with my partners Ryan, James, Haakon, Bryan, Dominic and the guidance of Allyson and John Lewis, we met each Monday at 1:00 p.m. in order to determine the length of this important unit. From our headquarters on top of the Science Center, we conducted 3 experiments in order to determine the angular diameter of the Sun, the rotational speed of the Sun, and the rotational period of the Sun. Using these values, we were then able to calculate the value of the Astronomical Unit. This is the first of 4 posts detailing our endeavors and today I will focus on our first experiment: Calculating the Angular Diameter of the Sun.

The Astronomical Unit

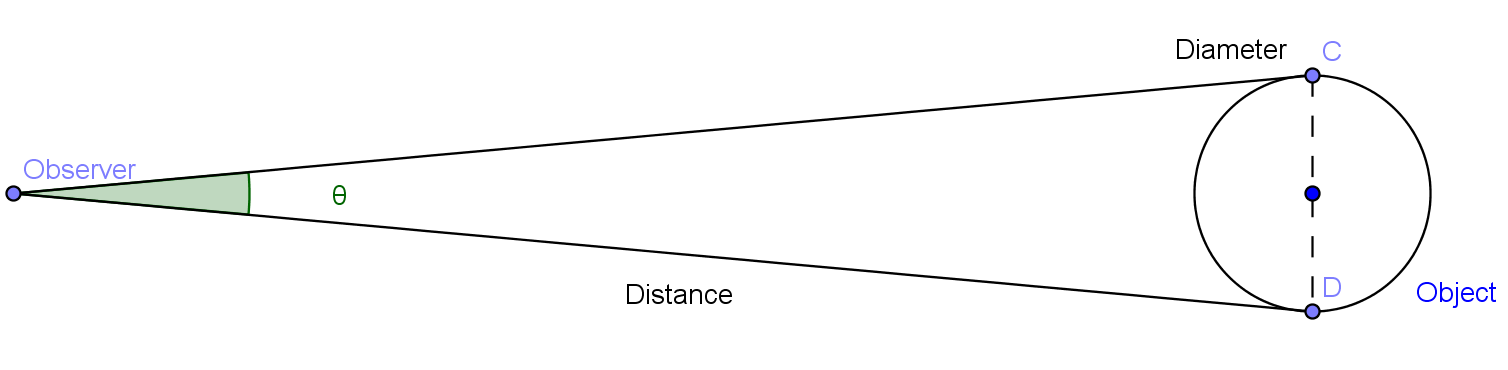
 The angular diameter shows the apparent size of an observed object with respect to the viewer. In the diagram below, angular diameter is represented by the angle \(\theta\). 

In order to determine this angular diameter, we used a system of lenses and mirrors to focus the light from the sun onto a wooden board in the form of a circle. We then measured the time that it took for this circle to move exactly one of its diameters horizontally. Here are the results from our trials:

Trial 1\(\:=\:00:02:10.59\:=\:\)130.59 seconds
Trial 2\(\:=\:00:02:20.00\:=\:\)140.00 seconds
Trial 3\(\:=\:00:02:18.07\:=\:\)138.07 seconds
Trial 4\(\:=\:00:02:18.06\:=\:\)138.06 seconds

The average time for the circle of light to move its own diameter was about 137 seconds.

Since we knew that the Earth takes 24 hours to complete one rotation, we could use this average time to set up a proportion and calculate the angular diameter of the Sun by seeing what fraction of the total rotation time (360 degrees) it takes to move one diameter (\(\theta\)).

\[\frac{\theta}{Avg\:Time}\:=\:\frac{360^{\circ}}{24\: hrs}\]
\[\theta\:=\:\frac{360^{\circ}\:\times\:Avg\:Time}{24\:hrs}\]
\[\theta\:=\:frac{{360^{\circ}\:\times\:137\:s}{86400\:s}\]
\[\theta\:\approx\:0.567^{\circ}\]

Thus the angular diameter of the sun was calculated to be about 0.567 degrees.